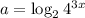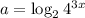
1) In this logarithm we can notice there are two variables. Let's rewrite it by applying the definition for Logarithm:
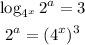
2) Now to solve for a, we need to perform some logarithm manipulation:
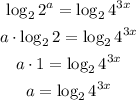
Note here, the application of logarithm of a power "dropping" the exponent in front of the logarithm expression and rewriting it as a factor.
3) Hence, the answer is: