Question

A 0.20 m long metal wire has a radius of 1 cm and a resistance of 3.2x10-5 Ω. What is the resistivity of the metal?Group of answer choices5.0x10-8 Ωm16x10-8 Ωm1.6x10-8 Ωm160x10-8 Ωm

Answer 1

Given that the length of the wire is l = 0.2 m

The radius of the wire is r = 1 cm = 0.01 m

The resistance of the wire is

`R\text{ = 3.2}*10^(-5)\Omega`

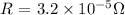
We have to find the resistivity of the metal.

Resistivity can be calculated by the formula,

`\begin{gathered} \rho=\text{ }\frac{Resis\tan ce\text{ }* Area}{length} \\ =(R\pi r^2)/(l) \end{gathered}`

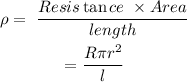
Substituting the values, the resistivity will be

`\begin{gathered} \rho\text{ =}(3.2*10^(-5)*\pi*(0.01)^2)/(0.2) \\ =5.0\text{ }*10^(-8)\Omega\text{ m} \end{gathered}`

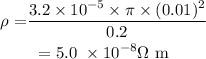
Thus, the resistivity of the metal is 5.0 x 10^(-8) ohm-meter.