Question

Find an equation for the perpendicular bisector of the line segment whose endpoints are (−2,−1) and ( − 6 , − 5 ).

Answer 1

`y = -x - 7`.

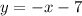Explanation:

Slope of the given line segment:

`\begin{aligned} m_(1) = ((-5) - (-1))/((-6) - (-2)) = 1\end{aligned}`.

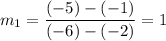The slope of any line perpendicular to this line segment would be:

`\begin{aligned}m_(2) &= (-1)/(m_(1)) = -1\end{aligned}`.

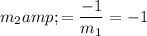Midpoint of the given line segment:

`\displaystyle \left(((-2) + (-6))/(2),\, ((-1) + (-5))/(2)\right)`.

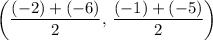Simplifies to get:

`(-4,\, -3)`.

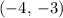Find the equation of the perpendicular bisector in point-slope form and simplify.

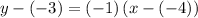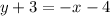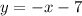`y - (-3) = (-1)\, (x - (-4))`.

`y + 3 = -x - 4`.

`y = -x - 7`.