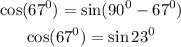Using trigonometry ratios SOH, CAH, TOA
Opposite (O) is the side facing the chosen angle, Adjacent is the side that is adjacent to the opposite side, and Hypothenuse (H) is the longest side facing the right angle

From the given right-angled triangle, with reference angle T
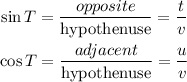

Part 2:
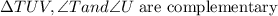
Complementary angles are angles that add up to 90°
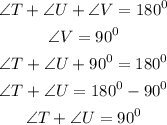
Since Part 3
From the trigonometric ratios gotten earlier above, it can be seen that

The second and the third options are true statements
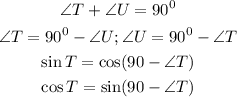
Part 4