Recall that in this method, we add two terms with the same variable, but with opposite coefficients, so that the sum is zero. In our case, both equations are already set equal to a constant. Notice that the coefficient of y in the second equation, –1, is the opposite of the coefficient of y in the first equation, 1. We can add the two equations to eliminate y without needing to multiply by a constant.
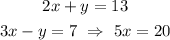
Now that we have eliminated y, we can solve the resulting equation for x

Then, we substitute this value for x into one of the original equations and solve for y.
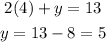
Thus, The solution to this system is (4,5)