Take into account that:

Then, for the given sine value, you can write:
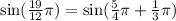
Now, consider that the sine of a sum is:

Then, by applying the previous identity to the given expression, you obtain:
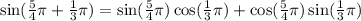
Consider now that:
![\begin{gathered} \sin ((5)/(4)\pi)=-\frac{\sqrt[]{2}}{2} \\ \cos ((1)/(3)\pi)=(1)/(2) \\ \sin ((1)/(3)\pi)=\frac{\sqrt[]{3}}{2} \\ \cos ((5)/(4)\pi)=-\frac{\sqrt[]{2}}{2} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/s9g5l0lvbu2dffubtgko8s6vyprk8baer7.png)
Then, for the given expression of the question, you get:
![\begin{gathered} \sin ((5)/(4)\pi+(1)/(3)\pi)=(-\frac{\sqrt[]{2}}{2})((1)/(2))+(-\frac{\sqrt[]{2}}{2})(\frac{\sqrt[]{3}}{2}) \\ =-\frac{\sqrt[]{2}}{4}-\frac{\sqrt[]{2}\sqrt[]{3}}{4}=-\frac{(1+\sqrt[]{3})\sqrt[]{2}}{4} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/vdmcc0gxqje4mfv7413326x7kq5nq9psi4.png)
The pervious result is the answer to the given expression.