Given:
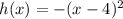
Let's use the transformations of the graph f(x) = x² to determine the function.
We have the transformation:
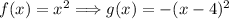
Simplify the transformed function:

Here, the following transformation occured:
• A horizontal translation right 4 units
,
• A reflection over the x-axis
Therefore, the graph of the function is below: