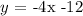Question:
Write the equation of a line passing through the points (-5, 8) and (-4, 4) in slope-Intercept form.
Solution:
The slope-intercept form of the line is given by the equation:
y = mx +b
where m is the slope of the line and b is the y-coordinate of the y-intercept (when x=0). Now, by definition the slope of the line is given by the following equation:
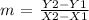
where (X1,Y1) and (X2,Y2) are points on the line. In this case, we have that
(X1, Y1) = (-5,8)
(X2,Y2) = (-4,4)
then, replacing these values in the equation of the slope we obtain:
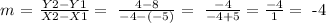
Thus, the equation of this line would be:
y = -4x +b
now, to find b, replace any point (x,y) in the above equation and solve for b. For example, take (x,y) = (-5,8). Then, we have:
8 = -4(-5) + b
this is equivalent to
8 = 20 + b
solve for b:
b = 8-20 = -12
thus, we can conclude that the equation in slope-intercept form of the given line is: