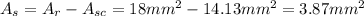Q) In the picture we have a semi-circle inscribed in a rectangle. We see that the diameter is equal to the width of the rectangle (the horizontal side) of the rectangle (w), so we have:
![d=w=6\operatorname{mm}]()
From the fact that the diameter is always two times the radius for every circle, we have:
![r=(d)/(2)=(6)/(2)\operatorname{mm}=3\operatorname{mm}]()
Now, we also see from the picture:
![h=r=3\operatorname{mm}]()
The question asks us about the area of the shaded region.
A) The shaded region can be computed in the following way:
1) First, we compute the area of the rectangle (Ar).
![A_r=w\cdot h=6\operatorname{mm}\cdot3\operatorname{mm}=18mm^2]()
2) Secondly, we compute the area of the semi-circle (Asc), which is half of the area of the entire circle.
![A_(sc)=(1)/(2)\cdot A_c=(1)/(2)\cdot\pi\cdot r^2=(1)/(2)\cdot3.14\cdot(3\operatorname{mm})^2=14.13mm^2]()
3) Finally, we compute the area of the shaded region taking the difference between the area of the rectangle and the area of the semi-circle.