Question

Use the properties of exponents to rewrite the expression. -5y² - 5y² - 5y² - 5y²A.-(5y²)4B. (-5y²)4C.-5y8D. none of theseReset Selection

Answer 1

Given the expression:

`-5y^2\cdot5y^2\cdot5y^2\cdot5y^2`

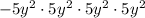
You need to apply the Product of Powers Property, which states that:

`b^m\cdot b^n=b^(m+n)`

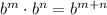
Then, you can add the exponents of the same base "y".

Notice that 5 is repeated 4 times. Therefore, you can rewrite the expression as follows:

`=-(5y^(2+2+2+2))`
`=-(5y^8)`

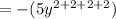
According to the Power of a Power Property:

`(b^m)^n=b^(mn)`

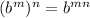
Therefore, you can rewrite the expression using that property:

`=-(5y^2)^4`

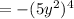
Hence, the answer is: Option A.