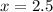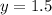Question

A store is having a sale on trail mix and jelly beans. For 5 pounds of trail mix and 3 pounds of jelly beans, the total cost is $17. For 2 pounds of trail mix and 12 pounds of jelly beans, the total cost is $23. Find the cost for each pound of trail mix and each pound of jelly beans.

Answer 1

Trail Mix costs $2.50 per pound, and Jelly Beans cost $1.50 per pound.

Explanation:

Using x for trail mix and y for jelly beans, we have a system of equations being

`\left \{ {{5x+3y=17} \atop {2x+12y=23}} \right.`

We can solve this system using substitution

5x+3y=17

Divide by 5 to have a coefficient of 1 before x

`x+(3)/(5) y=(17)/(5)`

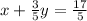
Subtract
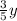`(3)/(5) y` from each side to single out one variable to one side

`x=(17)/(5) -(3)/(5) y`

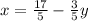
Now, we can plug in our value for x into the given equation

`2((17)/(5) -(3)/(5) y)+12y=23`

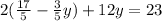
Open the parentheses

`(34)/(5) -1(1)/(5) y+12y=23`

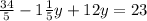
Merge Y values

`6.8+10.8y=23`

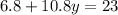
Separate numerical values from variables

`10.8y=16.2`

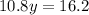
Divide by 10.8 in order to have a coefficient of 1

`y=1.5`

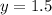
Now that we have one of the values, we know that a pound of jelly beans costs $1.50. We can now plug in 1.5 for y into one of our equations to find x.

`5x+3(1.5)=17`

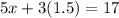
Open parentheses

`5x+4.5=17`

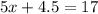
Single out the variable

`5x=12.5`

Divide by 5 to have a coefficient of 1 before x

`x=2.5`

`y=1.5`