Question

gr. 12 calcDetermine an expression for the area of a rectangle inscribed in a circle of radius 1 cm, in terms of angle θ as shown in the diagram below and be sure to state any restrictions on θ

Answer 1

From the diagram below

From the diagram, we have the hypotenuse of triagle ABD as 1 + 1 = 2cm

So using SOHCAHTOA, we have

`\begin{gathered} sin\varnothing=(oppsite)/(hypotenuse) \\ sin\varnothing=(x)/(2) \\ x=2sin\varnothing \\ Also\text{ } \\ cos\varnothing=(adjacent)/(hypotenuse) \\ cos\varnothing=(y)/(2) \\ y=2cos\varnothing \end{gathered}`

hence the area of the inscribed rectangle becomes

`\begin{gathered} Area=x* y \\ Area=(2sin\varnothing)(2cos\varnothing) \\ =4sin\varnothing cos\varnothing \end{gathered}`

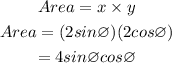
Hence the answer is

`Area=4sin\varnothing cos\varnothing`

(b) To get the maximum area, we find the derivative of the function above, we have

`\begin{gathered} A=4sin\varnothing cos\varnothing \\ simplify,\text{ we have } \\ 2sin(2\varnothing) \\ =(d)/(d\varnothing)(2sin(2\varnothing)) \\ take\text{ the constant out } \\ =2(d)/(d\varnothing)(sin(2\varnothing)) \end{gathered}`

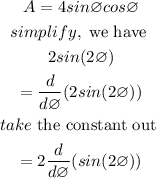
Apply the chain rule

`\begin{gathered} cos(2\varnothing)(d)/(d\varnothing)(2\varnothing) \\ =(d)/(d\varnothing)(2\varnothing)=2 \\ =2cos(2\varnothing)*2 \\ =4cos(2\varnothing) \end{gathered}`

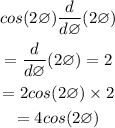
At maximum the derivative is 0, equating to zero, we have

`\begin{gathered} 4cos(2\varnothing)=0 \\ \varnothing=45\degree \end{gathered}`

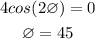
So, we put this 45 into the expression for area, we have

`\begin{gathered} Area=4sin\varnothing cos\varnothing \\ =4sin45\degree cos45\degree \\ =4*(√(2))/(2)*(√(2))/(2) \\ =(4*2)/(4) \\ =2units^2 \end{gathered}`

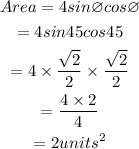
Hence the answer is 2 square units