Question

Find the height of the triangular pyramid when the volume is 318 sq

Answer 1

The rule of the volume of the triangular pyramid is

`V=(1)/(3)*(1)/(2)* b* h* H`

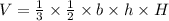
b is the base of the triangular base

h is the height of the triangular base

H is the height of the pyramid

From the given figure we can see

The base of the triangular base is 29 cm and its height is 9 cm, then

b = 29

h = 9

Since the volume of the pyramid is 318 cubic cm, then

V= 318

Substitute them in the rule above to find H

`\begin{gathered} 318=(1)/(3)*(1)/(2)*29*9* h \\ \\ 318=43.5H \\ \\ (318)/(43.5)=H \\ \\ 7.31=H \end{gathered}`

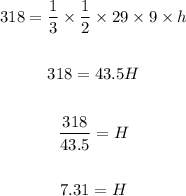
The height of the pyramid is 7.31 cm

The answer is A