Question

Helpppppppppppppp plssssss

Answer 1

D

Explanation:

Think of how you might express a line in a formula, there are many ways to do it but the only form the answers have is in the slope intercept form or

y=mx + b

Only B, D, and E fit this form so A and C can now be eliminated.

where m is the slope of a line and b is the y intercept of the line.

Here we see that the y intercept (or where the line hits the y-axis) is 0 so it should not have any constant b. This means it should be of the form

y = mx.

Finally, to find the slope m we use the rise over run of the slope of the line which can be put algebraically as

`(y_2-y_1)/(x_2-x_1)`

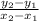
Where two points (x2, y2) and (x1, y1) are essentially being subtracted to give a slope. x2 and y2 are to the right of x1 and y1 to give the slope going from left to right.

On the graph we can see that the points with whole numbers are (0,0) (3,2), and (4,6). So we know that our answer must have x2 y2 and x1 y1 as whole numbers because those are the only options in our answers.

Although B uses the points (3,2) and (4,6) it subtracts them backwards with (3,2) as (x2,y2) instead of them acting as (x1,y1). This gives the slope from right to left which we do not want.

E puts x in the top part of the equation in the form
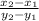`(x_2-x_1)/(y_2-y_1)` which is not the formula used to find slope which is why E is incorrect.

From this, we figure out that the only answer that has one of the options of the form
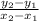`(y_2-y_1)/(x_2-x_1)` is answer D because it has the points (4,6) and (0,0) in the right order.