The line g(x), perpendicular to f(x), has a slope that is the negative reciprocal of the slope of f(x).
Since the slope of f(x) is -1/4, then the slope of g(x) is 4. Also, since g(x) passes through the origin (0, 0), then its equation is:
g(x) = 4x
So, those two lines intercept each other when
f(x) = g(x)
(-1/4)x + 8 = 4x
8 = 4x + x/4
8 = (16x + x)4
4 * 8 = 17x
32 = 17x
x = 32/17
Now, notice that f(x) has a y-intercept equal to 8. So, it intercepts the y-axis at y = 8, as shown below:
Now, we can find the area A of this triangle multiplying the side measuring 8 by the altitude of the triangle relative to that side, which is 32/17, and then divide it by 2:
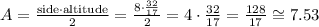
Therefore, the area is approximately 7.53.