Answer:
2.25
Step-by-step explanation:
The expected value can be calculated as:
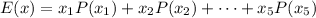
Where x1, x2, x3, x4, and x5 are the different values that the variable x can take and P(x1), P(x2), P(x3), P(x4), P(x5) are their respective probabilities.
Therefore, the expected value will be equal to:

So, the answer is 2.25