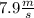1 Answer

Ask a Question

Steven Obua · Answer 1 · 2023-02-09T06:50:12+0000

The frequency of the waves is 17.5 Hz, and their wavelength is 45cm. Recall the following reation between the frequency, the wavelength and the speed of a wave:

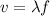
$v=\lambda f$

Substitute f=17.5Hz and λ=45cm to find the speed of the wave:

$\begin{gathered} v=(45\operatorname{cm})(17.5Hz) \\ =787.5\frac{\operatorname{cm}}{s} \\ =7.875(m)/(s) \\ \approx7.9(m)/(s) \end{gathered}$

Therefore, the speed of the waves is:

0 Comments

Please log in or register to add a comment.

Please log in or register to answer this question.

1 Answer

0 Comments

Please log in or register to add a comment.

Other Questions