given the following information
Distance= 180km
Speed first trip (S1)= S km/h
Speed on the return trip (S2)= S+9 km/h
the time first trip (t1) = t h
the time in the retunr trip ( t2)= t -1 h
since speed=D/t
we have the following equations
Eq1
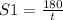
and
Eq2
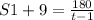
notice we have 2 equations and 2 variables
then Using eq1 in eq 2

solving for t


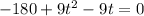
applying quadratic Formula
t=5 and t=-4
then
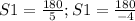
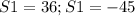
notice the speed A=36, B=-45
a negative symbol in speed represents direction
wich makes sense due to Jake is driving back home
then
the speed going is
S1=36
the speed returning is
S2=36+9=45