We have to prove that the tangent is an odd function.
If the tangent is an odd function, the following condition should be satisfied:

From the figure we can see that the tangent can be expressed as:
We can start then from tan(t) and will try to arrive to -tan(-t):
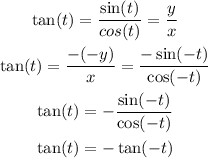
We have arrived to the condition for odd functions, so we have just proved that the tangent function is an odd function.