To find the graph of the first and the second function, let's substitute x by 1 and find the function that has the corresponding y value.
a)
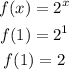
The graph that has the point (1, 2) is the green graph.
b)
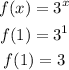
The graph that has the point (1, 3) is the yellow graph.
To find the graph of the third and the fourth function, let's substitute x by -1 and find the function that has the corresponding y value.
c)
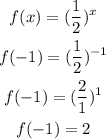
The graph that has the point (-1, 2) is the blue graph.
d)

The graph that has the point (-1, 3) is the red graph.
Answer:
![\begin{gathered} f(x)=2^x\Rightarrow g \\ f(x)=3^x\operatorname{\Rightarrow}y \\ f(x)=((1)/(2))^x\operatorname{\Rightarrow}b \\ f(x)=((1)/(3))^x\operatorname{\Rightarrow}r \end{gathered}]()