Solution:
Let A represent brand A, and B represent brand B. This implies that
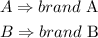
Given that A is 25% nuts and dried fruit, and B is 10% nuts and dried fruit, this implies that the total mixture is expressed as
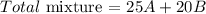
If A and B are mixed to form a 20 lb batch of sweet that is 13% nuts and dried fruit, thus implies that
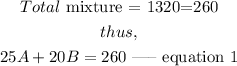
Also, given that A and B are mixed to form 20 lb batch, this implies that

To solve the amount of A and B, we solve the equations simultaneously.
Step 1: From equation 2, make B the subject of the equation.
Thus,
![undefined]()