If she ran 8 miles on the 3rd day and 35 miles on the 12th day, how far will she be able to run after 3 weeks?
Since Sarah increases her distance by the exact amount each day, the common difference is the same.
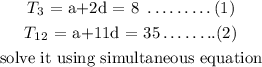
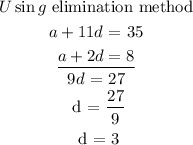
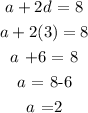
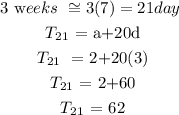
Hence she will be able to run after 3 weeks = 62 miles