The value of
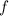 , rounded to the nearest hundredth, is approximately 0.35.
, rounded to the nearest hundredth, is approximately 0.35.
To determine the value of
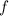 in the stopping distance formula provided in the image, we have the quadratic formula for stopping distance \( d \) as a function of velocity
in the stopping distance formula provided in the image, we have the quadratic formula for stopping distance \( d \) as a function of velocity
 :
:
![\[ d(v) = (2.15v^2)/(64.4f) \]](https://img.qammunity.org/2023/formulas/mathematics/college/bkc5dr7a97zo8yejsf43jtqe3nqjek9vn0.png)
We're given three pairs of
 and
and
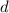 values in the table. We can substitute one pair into the equation to solve for
values in the table. We can substitute one pair into the equation to solve for
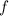 . It's typically best to use all the data points to find the most accurate value for
. It's typically best to use all the data points to find the most accurate value for
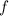 , but since we only need to determine
, but since we only need to determine
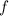 to the nearest hundredth, we can use any one of the data points provided.
to the nearest hundredth, we can use any one of the data points provided.
Let's use the pair
 and
and
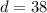 to find
to find
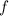 :
:
![\[ 38 = (2.15 \cdot 20^2)/(64.4f) \]](https://img.qammunity.org/2023/formulas/mathematics/college/11g5h0fjn5hc4kks9e4mw2h09yjnk73vj1.png)
Now, let's solve for
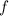 .
.
The value of
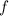 , rounded to the nearest hundredth, is approximately 0.35.
, rounded to the nearest hundredth, is approximately 0.35.