Question

Dan's family truck holds 14 gallons of fuel. Every day the car uses 0.6gallons of fuel. A warning light comes on when the remaining fuel is 1.5 gallons or less. Write and solve an equation to determine the number of days Dan's mother can drive the truck without the warning light coming on.

Answer 1

The number of gallons of fuel, Dan's truck can hold is

`=14`

The number of gallons of fuel Dan's truck can hold before a warning light showing is

`1.5\text{ gallons or less}`

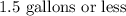
With this information, the number of gallons that will not show the warning light will be

`\begin{gathered} =14-1.5 \\ =12.5\text{ gallons or more} \end{gathered}`

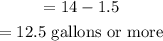
Let the number of days Dan will use the fuel without the warning light showing be

`=x`

The number of gallons per day is

`=0.6`

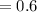
The number of gallons used in x-days will be

`0.6* x=0.6x`

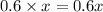
Hence,

The inequality to represent the situation will be

`0.6x<12.5`

Step 2:

To solve the number of days Dan's mother can drive the truck without the warning light showing, we will have to divide both sides by 0.6

`\begin{gathered} 0.6x<12.5 \\ (0.6x)/(0.6)<(12.5)/(0.6) \\ x<20.8 \end{gathered}`

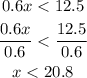
Hence,

The maximum number of days that Diego's father can drive the car without the warning light coming on is 20 days