Answer:
Step-by-step explanation:
To complete the missing values, we have to evaluate the function at the x-values given.
For example, to find the number below x = -1, we will put in x = -1 into y = (1/6)^x.
Putting in x = - 1 in y = (1/6)^x gives
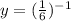
which simplifies to give

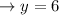
Similarly, for the missing value below x = -2, we put in x = - 2 into y = (1/6)^x to get
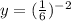
which simplifies to give
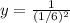

Similarly, for the missing value below x = 2, we put in x = 2 in y = (1/6)^x to get

which simplifies to give
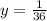
Hence, the missing value to be put in is 36.
To summerise, the missing values are
x | -2