Question

Given f = (1, -3) and g = (2,-3), find fg

Answer 1

We have to find the distance between F=(1, -3) and G=(2, -3).

The expression for the distance of points P1=(x1, y1) and P2=(x2,y2) is:

`D=√((y_1-y_2)^2+(x_1-x_2)^2)`

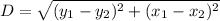
Then, if we replace with the coordinates of F and G, we have:

`\begin{gathered} D=√((-3-(-3))^2+(1-2)^2) \\ D=√(0^2+(-1)^2) \\ D=√(0+1) \\ D=√(1) \\ D=1 \end{gathered}`

The distance between F and G is 1 unit.

You can see it graphically as there is only one unit of difference in the x-axis and 0 units of difference in the y-axis.