1 Answer

EdgeCase · Answer 1 · 2022-10-12T15:26:05+0000

Answer:

h(-2) = 32

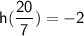
$\displaystyle\mathsf{h((20)/(7))=-2}$

Explanation:

Given the linear function, h(x) = -7x + 18:

h(-2)

In order to evaluate h(-2), simply substitute the value of the given input, x = -2, into the function.

h(x) = -7x + 18

h(-2) = -7(-2) + 18

h(-2) = 14 + 18

h(-2) = 32

Therefore, the output value when x = -2 is: h(-2) = 32.

This particular problem asks us to find the corresponding domain (x-value) given the range value of y = -2.

Substitute the value of y = -2 into the function to solve for the value of x:

h(x) = -7x + 18

-2 = -7x + 18

Subtract 18 from both sides:

-2 - 18 = -7x + 18 - 18

-20 = -7x

Divide both sides by -7 to solve for x:

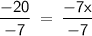
$\displaystyle\mathsf{(-20)/(-7)\:=\:(-7x)/(-7) }$

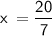
$\displaystyle\mathsf{x\:=(20)/(7)}$

Therefore, the value of x when h(x) = -2 is:
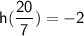$\displaystyle\mathsf{h((20)/(7))=-2}$ .