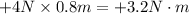Question

How do I resolve moments about the point P?

​

Answer 1

By applying the definition of torques (
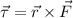`\vec \tau = \vec r * \vec F` ) and them remembering a few tricks.

Namely: if you wrap your RIGHT hand fingers around something and stick your thumb out, the direction your finger wraps gives you the verse of rotation and the thumb the orientation of the torque. Bottom force (4N) will give a counterclockwise rotation, torque is pointing up; top force (3N) will give a clockwise rotation and its torque its pointing down (read up and down as if the sheet the image is printed on is on your table).

In terms of magnitude the trick is easy: You want to multiply the intensity of the force (3N and 4N) by the distance between the point and the line the force it is applied to (that is, you don't care about the length of r itself, but the distance at a right angle, which is 0.9 and 0.8m respectively.

At this point, assuming "upwards" (relative to the plane of the sheet that is) torques positive, the 3N force gives you a torque of
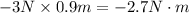`- 3N * 0.9m = - 2.7N\cdot m` and the 4N force provides
`+4N* 0.8 m = +3.2 N\cdot m`