We need to calculate the surface area of the square pyramid
In a square pyramid we 5 faces one is a square and the other for are triangles
for the area of the square
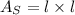
l=6.7 ft
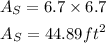
for the area of one triangle
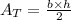
where
b=6.7ft
h=6 ft
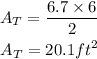
The surface area is
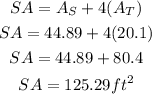
He needs 125.29 ft^2 of glass to cover the entire pyramid