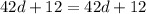Question

Use the Distributive Property to write and equivalent expression.Problem 1:6(h + 3.5) = ___Problem 2:5m + 5p = ___Problem 3: 2.5(w + 3) = ___Problem 4:5(3z + 4) = ___Problem 5:42d + 12 = ___

Answer 1

The distributive property tells us that when we have a number or term that multiplies an expression grouped by parenthesis, we need to multiply the number outside the parenthesis by the numbers or terms inside the parenthesis.

Problem 1:

`6\mleft(h+3.5\mright)`

Using the distributive property we get:

`6(h+3.5)=6\cdot h+6\cdot3.5`

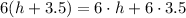
Solving the multiplications: 6 times h is 6h, and 6 times 3.5 is 21, so the result is:

`6(h+3.5)=6h+21`

Problem 2:

`5m+5p`

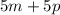
We can't use the distributive property because we don't have a multiplication by a group of terms, nor we have like terms to combine, so the result is the same expression:

`5m+5p=5m+5p`

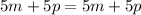
Problem 3:

`2.5\mleft(w+3\mright)`

Using the distributive property:

`2.5\mleft(w+3\mright)=2.5\cdot w+2.5\cdot3`

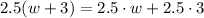
Solving the multiplications: 2.5 times w is 2.5w, and 2.5 times 3 is 7.5. The result is:

`2.5\mleft(w+3\mright)=2.5w+7.5`

Problem 4:

`5\mleft(3z+4\mright)`

Using the distributive property:

`5\mleft(3z+4\mright)=5\cdot3z+5\cdot4`

Solving the multiplications: 5 times 3z is equal to 15z, and 5 times 4 is 20. So the result is:

`5\mleft(3z+4\mright)=15z+20`

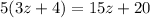
Problem 5:

`42d+12`

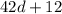
We have something similar that we had in problem 2, there are no grouped terms to multiply by an outside term, so we cannot use the distributive property here, and the result is the same expression:

`42d+12=42d+12`