The slope of the equation in the form a x + by = c is
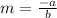
Where a is the coefficient of x and b is the coefficient of y
∵ The equation is 2x + 3y = 4
∴ a = 2 and b = 3
Substitute them in the rule above
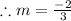
The slope of the line is -2/3
The product of the slopes of the perpendicular lines is 1
That means if the slope of one is m, then the slope of the other is -1/m
To find the slope of the perpendicular line, we will reciprocal it and change its sign
The slope of the perpendicular line to the given line is
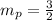
The slope of the perpendicular line is 3/2