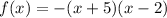You have to factor the following function
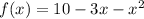
The coefficients of the function are:
a=-1
b=-3
c=10
Using the quadratic formula you have to calculate the roots of the function
![x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}](https://img.qammunity.org/2023/formulas/mathematics/college/rxvf73usjbbwyik14knxdemoz21vfz2ufc.png)
Replace the formula with the values of the coefficients of the function
![\begin{gathered} x=\frac{-(-3)\pm\sqrt[]{(-3)^2-4(-1)10}}{2(-1)} \\ x=\frac{3\pm\sqrt[]{9+40}}{-2} \\ x=\frac{3\pm\sqrt[]{49}}{-2} \\ x=(3\pm7)/(-2) \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/high-school/z5ae7q4uqbk7i8z7v0mlewi9vhgggxdtt4.png)
Positive calculation:
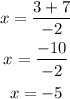
Negative calculation

The roots of the function are x=-5 and x=2
The factorized function is
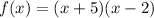
Note that the roots have to have the inverse sign when you write the factorized function.
The final step is to multiply the function by -1, to make it point downwards just like the original one.