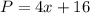To find the perimeter in terms of x, follow the steps below.
Step 01: Factor out the quadratic equation.
To do it, find its roots using the quadratic formula.
For a quadratic equation y = ax² + bx + c, the quadratic formula is:
![x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}](https://img.qammunity.org/2023/formulas/mathematics/college/rxvf73usjbbwyik14knxdemoz21vfz2ufc.png)
In this question:
a = 1
b = 8
c = 16
Then:
![\begin{gathered} x=\frac{-8\pm\sqrt[]{8^2-4\cdot1\cdot16}}{2\cdot1} \\ x=\frac{-8\pm\sqrt[]{64-64}}{2} \\ x=\frac{-8\pm\sqrt[]{0}}{2} \\ x_1=(-8-0)/(2)=-(8)/(2)=-4 \\ x_2=(-8+0)/(2)=-(8)/(2)=-4 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/wppurg56awbhwv71xqcmda4hdsa9x189v8.png)
A quadratic equation in the factored form is y = (x - x₁)(x - x₂), where x₁ and x₂ are the roots.
Then, the quadratic equation can be written as:
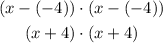
Step 02: Find the sides of the rectangle.
The area of the rectangle is:
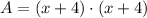
A rectangle with sides "a" and "b" has an area (A):
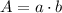
Then, the sides of the triangle are:
a = x + 4
b = x + 4
Step 03: Find the perimeter.
A rectangle with sides "a" and "b" has the perimeter (P):
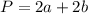
Since:
a = x + 4
b = x + 4
Then,
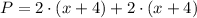
Solving the equation:
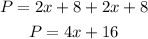
Answer:
The perimeter (P) is: