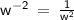Question

W^3 x w^-5
please help and please explain

Answer 1

= W^3+(-5)
= W^3-5
= W^-2

Answer 2

`\Large \text{$ (1)/(w^2) $}`

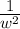
Explanation:

Given the exponential expression,
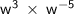`\displaytext\mathsf{w^3\:*\:w^(-5)}`, where it involves the multiplication of the same base, w, with varying powers.

Using the Product Rule of Exponents, where it states that,
`\displaystyle\mathsf{a^(m)\:*\:a^(n)\:=\:a^((m\:+\:n))}`.

Hence, we simply need to add the exponents:

`\displaytext\mathsf{w^3\:*\:w^(-5)\:=\:w\:^([3\:+\:(-5)])\:=\:w^(-2)}`

Next, apply the Negative Exponent Rule, where it states that:
`\displaystyle\mathsf{a^(-n)\:=\:(1)/(a^n)}`.

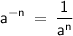Transforming the negative exponent of
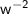`\displaytext\mathsf{w^(-2)}` becomes a positive exponent by using the Negative Exponent Rule.

`\displaytext\mathsf{w^(-2)\:=\:(1)/(w^2)}`