Given the following equation:
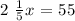
Let's determine the value of x.
Step 1: Let's first transform 2 1/5 into an improper fraction.
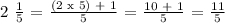
Step 2: Let's simplify.
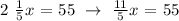
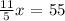
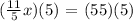
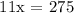
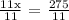
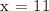
Therefore, x = 11
Keep in mind that for an equation with a constant that is a mixed number. Always transform it first into an improper fraction and apply the inverse property in simplifying or determining the value of x.