Question

A crate of mass 70 kg is pulled a distance of 12 m up an inclined plane and in the process it is raised 2.0 m as shown in diagram below. In order to do this, a force of 150 N is applied to the crate in a direction parallel to the inclined plane.(i) what is the gtavitational potential energy output?(ii) What is the work done by the force aka work input?(iii) What is the efficiency of the system?(iv) Why are the answers fo 12 (i) and (ii) different?

Answer 1

(i) 1400 J

(ii) 1800 J

(iii) 77.78%

(iv) Between two answers are different because the first work is done by an external agent 150 N while the increase in potential energy is the work done by the gravitational force

Explanation:

Given:

Mass (m) = 70 kg

Height (h) = 2 m

Distance (d) = 12 m

Force (F) = 150 N

(i)

We calculate the gravitational potential energy using the following formula:

`\begin{gathered} E_P=mgh \\ \\ \text{ We replacing} \\ \\ E_P=70\cdot10\cdot2 \\ \\ E_P=1400\text{ J} \end{gathered}`

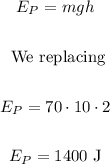
(ii)

We calculate the force as follows:

`\begin{gathered} W=F\cdot d \\ \\ \text{ We replacing:} \\ \\ W=150\cdot12 \\ \\ W=1800\text{ J} \end{gathered}`

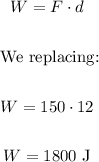
(iii)

The efficiency of the system can be calculated by means of the quotient between the output energy and the input energy, therefore:

`\begin{gathered} e=(E_(out))/(E_(in))\cdot100 \\ \\ \text{ We replacing:} \\ \\ e=(1400)/(1800) \\ \\ e=77.78\% \end{gathered}`

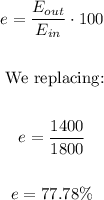
(iv)

Between two answers are different because the first work is done by an external agent 150 N while the increase in potential energy is the work done by the gravitational force