Question

To write an equation of a line that is...

parallel to 2x - 4y = 8
and goes through the point (3, -2)

Answer 1

x-2y=7 or y=
`(1)/(2)x-(7)/(2)`

Explanation:

Hi there!

We are given the line 2x-4y=8, and we want to write an equation of the line that is parallel to it and passes through (3, -2)

Parallel lines have the same slope, so it would be a good idea to find the slope of 2x-4y=8

The equation is currently written in standard form (ax+by=c), where a, b, and c are free integer coefficients, but a and b CANNOT be equal to 0, and a CANNOT be negative

In order to find the slope of the line, we can rewrite it in another form; for example, slope-intercept form (y=mx+b, where m is the slope and b is the y intercept)

To rewrite the line in this way, we'll need to isolate y on one side.

So start by subtracting 2x from both sides

-4y=-2x+8

Divide both sides by -4

y=
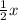`(1)/(2)x`-2

The slope of the line 1/2, as it's in the place of where m is.

It's also the slope of the line parallel to it.

We can write the equation of the new line in slope-intercept form. Here's what we know so far:

y=
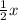`(1)/(2)x`+b (b is a placeholder for the y intercept)

So we'll need to find b.

As the equation passes through the point (3, -2), we can use it to solve for b

Substitute 3 as x and -2 as y:

-2=1/2(3)+b

Multiply

-2=3/2+b

Subtract 3/2 from both sides:

-7/2=b

Substitute -7/2 as b in the equation:

y=
`(1)/(2)x-(7)/(2)`

The equation can be left as that, or you can convert it back into standard form

Subtract
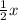`(1)/(2)x` from both sides, as the variables are on one side.

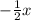`-(1)/(2)x`+y=
`-(7)/(2)`

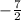
Remember that the coefficient in front of x (a) CANNOT be negative, and also the free coefficients a, b, and c CANNOT be fractions.

So in order to clear the fractions and to change the signs, multiply both sides by -2

x-2y=7

Hope this helps!