To find the value of y for point (-3,y), knowing that the line that links the said point and point (5,9) has a slope equal to 1, first, you have to determine the equation of the line.
For this, you have to use the point-slope form:

Where
m is the slope of the line
(x₁,y₁) are the coordinate of one point of the line
Replace the formula with x₁=5, y₁=9, and m=1
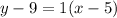
Now let's write the equation in slope-intercept form:
-Distribute the multiplication on the parentheses term:
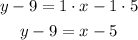
-Pass "-9" to the right side of the equal sign by applying the opposite operation "+9" to both sides of it:

Once the equation of the line is determined, replace it with x=-3 to calculate the corresponding y-value:
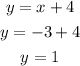
The value of y is 1, you can write the coordinate pair as (-3,1)