Question

Sarah thinks that the following expressions are equivalent: 2x + 3 = x/2+ ¾ Is she right? If so, prove that these two expressions are equivalent. If not, what error(s) did she make?

Answer 1

Given that Sarah thinks;

`2x+3=(x)/(2)+(3)/(4)`

ANSWER: The two expressions are not equivalent because;

`(x)/(2)+(3)/(4)=(1)/(4)(2x+3)`

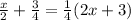
That is, 1/4 of the expression on the left side is on the right side.

Another Method

Let x =2, then substitute;

`\begin{gathered} 2x+3=2(2)+3 \\ \\ 2x+3=4+3 \\ \\ 2x+3=7 \end{gathered}`

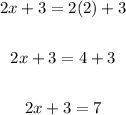
Then, substitute in the second expression too;

`\begin{gathered} (x)/(2)+(3)/(4)=(2)/(2)+(3)/(4) \\ \\ (x)/(2)+(3)/(4)=1+(3)/(4) \\ \\ (x)/(2)+(3)/(4)=(7)/(4) \end{gathered}`

Since, the answers are not the same, then, the expressions are not equivalent.