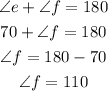1 Answer

Alexander McNulty · Answer 1 · 2023-08-03T17:09:45+0000

The angles ∠135° and ∠b are supplementary angles, so we have:

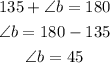
$\begin{gathered} 135+\angle b=180 \\ \angle b=180-135 \\ \angle b=45\degree \end{gathered}$

The angles ∠70° and ∠a are vertically opposite angles, so they are congruent:

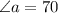
$\angle a=70\degree$

Using the sum of internal angles of the triangle equal 180°, we have:

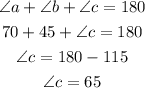
$\begin{gathered} \angle a+\angle b+\angle c=180 \\ 70+45+\angle c=180 \\ \angle c=180-115 \\ \angle c=65\degree \end{gathered}$

The angles ∠b and ∠d are alternate interior angles, so they are congruent:

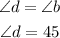
$\begin{gathered} \angle d=\angle b \\ \angle d=45\degree \end{gathered}$

The sum of ∠d, ∠c and ∠e is equal 180°, so we have:

$\begin{gathered} \angle d+\angle c+\angle e=180 \\ 45+65+\angle e=180 \\ \angle e=180-110 \\ \angle e=70\degree \end{gathered}$

The angles ∠e and ∠f are supplementary, so we have:

$\begin{gathered} \angle e+\angle f=180 \\ 70+\angle f=180 \\ \angle f=180-70 \\ \angle f=110\degree \end{gathered}$

Please log in or register to add a comment.