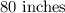A)
The area of a square is equal to the square of the length of its side. Since the side of the square is x, then the area is equal to x^2. Since the area must be equal to 400 square inches, then:
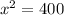
B)
To solve this equation, take the square root to both members of the equation:
![\Rightarrow\sqrt[]{x^2}=\sqrt[]{400}](https://img.qammunity.org/2023/formulas/mathematics/college/28zejh5498tu0l4lnj8ax9ef1t8zftael3.png)
The square root of the square of a variable is always equal to the absolute value of that variable. The square root of 400 is 20. Then:
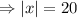
This equation has two solutions:

C)
Since x represents a distance, the negative solution does not make sense in the context of the problem.
D)
Since a square has four sides of equal lengths, and one side has a length of 20 inches, then four times 20 inches is the length needed to go around the painting. Since 4*20=80, then, the length of the wood trim needed to go around the painting, is: