Question

The population of the world in 1987 was 5 billion and the annual growth rate was estimated at 1.2% per year. Assuming that the world population follows an exponential growth model, find the projected world population in 2019. Round your answer to 1 decimal place.

Answer 1

Given:

Population of the world in 1987 = 5 billion

Annual growth rate = 1.2% per year.

We want to determine the projected world population in 2019.

An exponential growth can be modelled by the function

`\begin{gathered} f(x)=a(1+r)^x \\ Where\text{ a=initial population} \\ r=rate\text{ \lparen in \%\rparen} \\ x=\text{ period\lparen number of times\rparen} \end{gathered}`

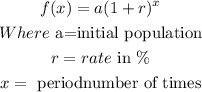
For the question before us,

r=1.2% = 0.012

x = 2019 -1987 = 32

a = 5,000,000,000

Thus,

`\begin{gathered} f(32)=5,000,000,000(1+0.012)^(32) \\ =5000000000(1.012)^(32) \\ =500000000(1.464793) \\ =7323967432.519 \\ =7323967432.5\text{ \lparen1 decimal place\rparen} \end{gathered}`

The projected world population in 2019 is 7,323,967,432.5