Question

Select the correct answerAnnabeth and Charlie are both on road trips. Annabeth's distance, in miles, from New York thours after 12:00p.m. is modeled by this function.D(t) = 60lt – 31Charlie's distance, in miles, from New York thours after 12:00 p.m. is modeled by this table,t0246810F(t)27018090090180Who will have traveled for a greater amount of time when their distance from New York stops decreasing and starts increasing?ОА.CharlieOBThis cannot be determined from the given informationOC. They will have traveled the same amount of time,ODAnnabeth

Answer 1

We are given that Annabeth's distance from New York is;

`D(t)=60t-31`

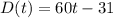
The graph of this equation is the following:

We notice that the distance stops decreasing and starts increasing where the value of the function is zero, therefore, we set the function to zero:

`60t-31=0`

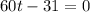
Now, we solve for "t". First, we add 31 to both sides:

`60t=31`

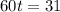
Now, we divide both sides by 60;

`\begin{gathered} t=(31)/(60) \\ \\ t=0.52 \end{gathered}`

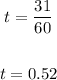
Therefore, the time for Annabeth is 0.52

Now, we determine the value for the function that models Charlie's distance. According to the table, the function for Charlie is zero at

`t=6`

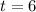
Therefore, Charlie will have traveled for a greater amount of time.