Question

A raft that has a density of 710 kg/m^3 is set in a lake of fresh water (density = 1000 kg/m3).The volume of the raft is 2.9 m3 and the area of the top of the raft is 5.6 m. What is the height h of the raft that is below the water line?

Answer 1

`0.368\text{ }m`

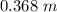
Step-by-step explanation

First, we have to find the mass of the raft.

To do this, apply the formula for density:

`\rho=(m)/(V)`

where m = mass of the raft; V = volume of the raft

Hence, solving for m, we have that the mass of the raft is:

`\begin{gathered} m=\rho *V \\ m=710*2.9 \\ m=2059\text{ }kg \end{gathered}`

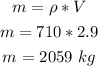
The mass of the raft is equal to the mass of water displaced by the raft. Hence, we can find the volume of water displaced by applying the formula for density:

`\begin{gathered} \rho=(m)/(V) \\ V=(m)/(\rho) \\ V=(2059)/(1000) \\ V=2.059\text{ }m^3 \end{gathered}`

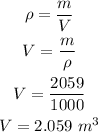
We now need to find the height of the water displaced This height is equal to the height of the box that is below the water line.

To do this, we can apply the formula for volume, since the area of the top of the raft is equal to the area of the water it covers:

`\begin{gathered} V=A*h \\ h=(V)/(A) \end{gathered}`

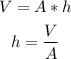
Hence, the height of the raft that is below the water line is:

`\begin{gathered} h=(2.059)/(5.6) \\ h=0.368\text{ }m \end{gathered}`

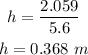
That is the height of the raft that is below the water line.