As we can se from the graph, the maximum value of the function is almost 15 meters, it is reached between t=1s and t=2s, and the height is 0 again when the time is approximately 3 seconds.
We can find the exact values by using the equation.
First, find the zeroes of the function by setting h(t)=0 and solving the quadratic equation for t:
![\begin{gathered} h(t)=-5t^2+13t+6=0 \\ \Rightarrow t=\frac{-13\pm\sqrt[]{13^2-4(6)(-5)}}{2(-5)} \\ \Rightarrow t_1=-0.4;t_2=3 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/dgln99z3cwus2z6um331kfs5cnzpb4m0eu.png)
Then, the projectile hits the ground at t=3s.
To find the time at which the projectile reaches its maximum height, find the average between both zeroes:
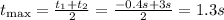
To find the maximum height, evaluate h at t=1.3s:
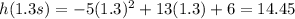
Therefore, to the nearest tenth, the projectile reached a maximum height of 14.5 meters in 1.3 seconds and it took 3.0 seconds for it to hit the ground.
The correct option is the second one.