Question

Find the range variance and standard deviation for the sample data

Answer 1

Answers:

Range = 25

Variance = 102.23

Standard deviation = 10.11

Step-by-step explanation:

The range of the data can be calculated as the difference between the greatest and smallest value, so the range is equal to:

Range = 39 - 14

Range = 25

Then, to find the variance, we need to find the mean. The mean is equal to the sum of all values divided by the total number of values. So:

Now, we need to complete the following table:

Value (Value - mean)²

39 (39-24.98)² = 196.56

38 (38-24.98)² = 169.52

37 (37-24.98)² = 144.48

30 (30-24.98)² = 25.2

22 (22-24.98)² = 8.88

21 (21-24.98)² = 15.84

19 (19-24.98)² = 35.76

15 (15-24.98)² = 99.6

14.8 (14.8-24.98)² = 103.63

14 (14-24.98)² = 120.56

Then, the variance will be equal to the sum of these numbers divided by the size of the sample less 1. So:

`\begin{gathered} \text{Variance = }(196.56+169.52+\cdots+99.6+103.63+120.56)/(10-1) \\ \text{Variance = }(920.036)/(9) \\ \text{Variance = }102.23 \end{gathered}`

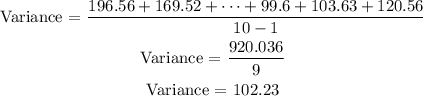
Finally, the standard deviation is the square root of the variance, so

`\begin{gathered} \text{ Standard deviation = }\sqrt[]{102.23} \\ \text{Standard deviation =}10.11 \end{gathered}`

We can't say anything about the variation in general because this vis the top 10 of annual salaries,