Given,
The equations of line are,
y = -1/6x + 2
y = 6x + 4
The standard form of equation of line is: y = mx+c
On comparing the equation of line to the standard equation then,
The slope of line is y = -1/6x + 2 is -1/6.
The slope of line is y = 6x + 2 is 6.
When the line are parallel then
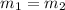
When the line are perpendicular then,
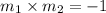
Substituting the value of slopes then,
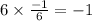
Hence, the product of the slope of the line is equal to -1. So, the line are perpendicular to each other.