Question

How many roots does x^2-6x+9 have ? It may help to graph the equation.

Answer 1

The roots are those values that make a function or polynomial take a zero value. The roots are also the intersection points with the x-axis. In the case of a quadratic equation you can use the quadratic formula to find its roots:

`\begin{gathered} ax^2+bx+c=y\Rightarrow\text{ Quadratic equation in standard form} \\ x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}\Rightarrow\text{ Quadratic formula} \end{gathered}`

So, in this case, you have

`\begin{gathered} y=x^2-6x+9 \\ a=1 \\ b=-6 \\ c=9 \end{gathered}`
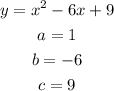
`\begin{gathered} x=\frac{-(-6)\pm\sqrt[]{(-6)^2-4(1)(9)}}{2(1)} \\ x=\frac{6\pm\sqrt[]{36-36}}{2} \\ x=(6\pm0)/(2) \\ x=(6)/(2) \\ x=3 \end{gathered}`

As you can see, this function only has one root, at x = 3.

You can see this in the graph of the function: