In order to determine the speed of the Earth, proceed as follow:
Consider that the centripetal force must be equal to the gravitational force between the Earth and the Sun (because guarantees the stability of the system):
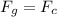
Fg is the gravitational force and Fc the centripetal force. The expressions for each of these forces are:
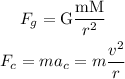
where,
G: Cavendish's constant = 6.67*10^-11 Nm^2/kg^2
m: Earth's mass = 5.97*10^24 kg
M: Sun's mass = 1.99*10^30kg
v: speed of Earth around the Sun = ?
r: distance between the center of mass of Earth and Sun = 1.49*10^8km = 1.49*10^11 m
Equal the expressions for Fg and Fc, solve for v, replace the previous values of the parameters and simplify:
![\begin{gathered} \text{G}\frac{\text{mM}}{r^2}=m(v^2)/(r) \\ v^{}=\sqrt[]{(GM)/(r)} \\ v=\sqrt[]{\frac{(6.67\cdot10^(-11)N\frac{m^2}{\operatorname{kg}^2})(1.99\cdot10^(30)kg)}{1.49\cdot10^(11)m}} \\ v\approx29846.7(m)/(s) \end{gathered}]()
Hence, the speed of the Earth around the Sun is approximately 29846.7m/s