SOLUTION
Given the question in the image, the following are the solution steps to answer the question.
STEP 1: Draw the given scenario
STEP 2: Describe how to answer the question
The question forms a right angle triangle. where the height of the cat on the tree is the opposite side of the triangle. The distance between the cat and the tree is the adjacent side of the triangle .
Recall the 95 foot ladder can only start 8 feet above the ground .The diagram is represented above:
The ladder height should be the hypotenuse of the triangle.
using Pythagoras's theorem,
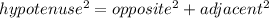
STEP 3: Write the given sides
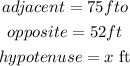
STEP 4: find x

The expected length of the ladder should be approximately 91.26ft. Since the ladder is 95 foot, therefore the cat will be rescued with the given ladder.